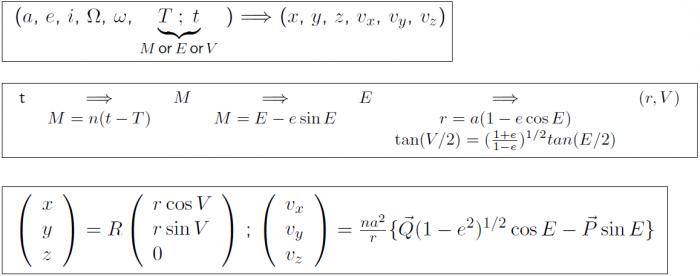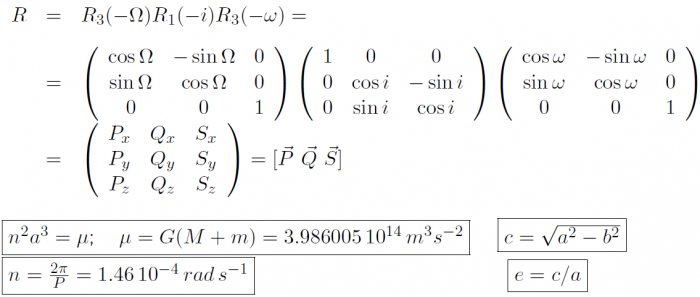If you wish to contribute or participate in the discussions about articles you are invited to contact the Editor
APPROVED
Not Approved
Vicente.Navarro approved this page 14 July
14 July
Osculating Elements
Jump to navigation
Jump to search
| Fundamentals | |
|---|---|
| Title | Osculating Elements |
| Author(s) | J. Sanz Subirana, J.M. Juan Zornoza and M. Hernández-Pajares, Technical University of Catalonia, Spain. |
| Level | Advanced |
| Year of Publication | 2011 |
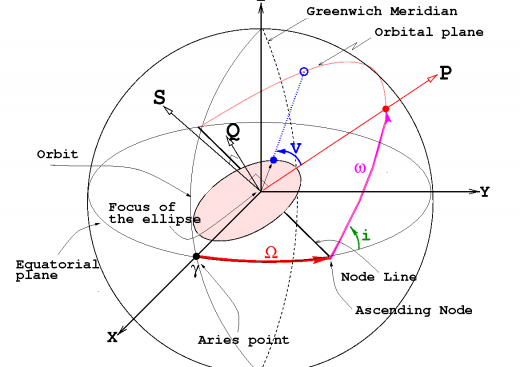
A scheme with the necessary calculations to obtain the osculating orbital elements starting from the position and velocity of a satellite in a geocentric inertial system, and vice-versa, is provided as follows (see figure 1):
Calculation of the orbital elements of the satellite from its position and velocity
- [math]\displaystyle{ (x, y, z, v_x, v_y, v_z) \Longrightarrow (a, e, i, \Omega, \omega, M) \qquad \mbox{(1)} }[/math]
- [math]\displaystyle{ \vec c= \vec r \times \vec v \Longrightarrow p=\frac{c^2}{\mu} \Longrightarrow p \qquad \mbox{(2)} }[/math]
- [math]\displaystyle{ v^2=\mu (2/r -1/a) \Longrightarrow a \qquad \mbox{(3)} }[/math]
- [math]\displaystyle{ p=a(1-e^2) \Longrightarrow e \qquad \mbox{(4)} }[/math]
- [math]\displaystyle{ \vec c = c \vec S \Longrightarrow \Omega=\arctan(-c_x/c_y); i=arcs(c_z/c) \Longrightarrow \Omega, i \qquad \mbox{(5)} }[/math]
- [math]\displaystyle{ \left( \begin{array}{l} x\\ y\\ z \end{array} \right) = R \left( \begin{array}{l} r \cos(V)\\ r \sin(V)\\ 0 \end{array} \right) = r \left( \begin{array}{l} \cos \Omega \cos(\omega+V)- \sin \Omega \sin(\omega+V) \cos i\\ \sin \Omega \cos(\omega+V)+ \cos \Omega \sin(\omega+V) \cos i\\ \sin(\omega+V) \sin i \end{array} \right) \Rightarrow \omega+V \qquad \mbox{(6)} }[/math]
- [math]\displaystyle{ r=\frac{p}{1+e\cos(V)} \Longrightarrow \omega, V \qquad \mbox{(7)} }[/math]
- [math]\displaystyle{ \tan(E/2)=(\frac{1-e}{1+e})^{1/2}\tan(V/2) \Longrightarrow E \qquad \mbox{(8)} }[/math]
- [math]\displaystyle{ M= E -e \sin E \Longrightarrow M \qquad \mbox{(9)} }[/math]
Calculation of the position and velocity of the satellite from its orbital elements
- (10)
- where
- (11)