If you wish to contribute or participate in the discussions about articles you are invited to contact the Editor
Relativistic Path Range Effect: Difference between revisions
Carlos.Lopez (talk | contribs) No edit summary |
Carlos.Lopez (talk | contribs) No edit summary |
||
| Line 17: | Line 17: | ||
where <math>r^{sat}, r_{rcv} </math> are the geocentric distances of satellite and receiver and <math> r_{rcv}^{sat} </math> is the distance between them. The constants <math>c = 299792458 m/s </math> and <math>c=299792458\,m/s </math> and <math>\mu=G\,M_\oplus=3.986004418 \cdot 10^{14}\, m^3/s^2 </math> are the sped of light and the | where <math>r^{sat}, r_{rcv} </math> are the geocentric distances of satellite and receiver and <math> r_{rcv}^{sat} </math> is the distance between them. The constants <math>c = 299792458 m/s </math> and <math>c=299792458\,m/s </math> and <math>\mu=G\,M_\oplus=3.986004418 \cdot 10^{14}\, m^3/s^2 </math> are the sped of light and the Earth's gravitational constant, respectively (see [[Reference Frames in GNSS]]). | ||
| Line 23: | Line 24: | ||
::<math> \rho_{rcv}^{sat}=\left\| {r}_{rcv}-{r}^{sat}\right \|=\sqrt{(x_{rcv}-x^{sat})^2+(y_{rcv}-y^{sat})^2+(z_{rcv}-z^{sat})^2} \qquad \mbox{(2)}</math> | ::<math> \rho_{rcv}^{sat}=\left\| {r}_{rcv}-{r}^{sat}\right \|=\sqrt{(x_{rcv}-x^{sat})^2+(y_{rcv}-y^{sat})^2+(z_{rcv}-z^{sat})^2} \qquad \mbox{(2)}</math> | ||
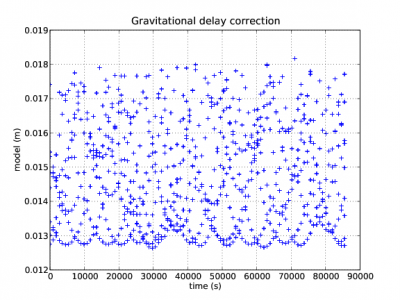
Next figure 1 illustrates with an example the Shapiro signal propagation delays for the satellites in view from a receiver in Barcelona, Spain (receiver coordinates <math> \lambda\simeq 2^ o $\phi \simeq 41^o</math>). | Next figure 1 illustrates with an example the Shapiro signal propagation delays for the satellites in view from a receiver in Barcelona, Spain (receiver coordinates <math> \lambda\simeq 2^ o $\phi \simeq 41^o</math>). | ||
A very good review of relativistic effects on GPS can be found in [Ashby, N., 2003], (see http://relativity.livingreviews.org/Articles/lrr-2003-1). | ::[[File:Geom_Range_Model_Relat_Path_Range_Effect.png|none|thumb|400px|'''''Figure 1:''''' Shapiro correction to the geometric range for the satellites in view from a receiver at <math> \lambda\simeq 2^ o $\phi \simeq 41^o</math> coordinates.]] | ||
A very good review of relativistic effects on GPS can be found in [Ashby, N., 2003] <ref>[Ashby, N., 2003] Ashby, N., 2003. Relativity in the Global Positioning System. http://relativity.livingreviews.org/articles/lrr-2003-1/.</ref> , (see http://relativity.livingreviews.org/Articles/lrr-2003-1). | |||
==References== | ==References== | ||
<references/> | |||
[[Category:Fundamentals]] | [[Category:Fundamentals]] | ||
Revision as of 09:28, 8 August 2011
| Fundamentals | |
|---|---|
| Title | Relativistic Path Range Effect |
| Author(s) | J. Sanz Subirana, JM. Juan Zornoza and M. Hernandez-Pajares, University of Catalunia, Spain. |
| Level | Advanced |
| Year of Publication | 2011 |
This is a secondary relativistic effect that can be required only for high accuracy positioning. Its net effect on range is less than 2 cm and thence, for most
purposes it can be neglected.
This effect is named the Shapiro signal propagation delay and introduces a general relativistic correction to the geometric range: Due to the space-time curvature produced by the gravitational field, the Euclidean range computed by carrier phase measurement must be corrected by an amount given by the expression:
- [math]\displaystyle{ \rho_{_{rel}}=\frac{2\,\mu}{c^2}ln\frac{r^{sat}+r_{rcv}+r_{rcv}^{sat}}{r^{sat}+r_{rcv}-r_{rcv}^{sat}} \qquad \mbox{(1)} }[/math]
where [math]\displaystyle{ r^{sat}, r_{rcv} }[/math] are the geocentric distances of satellite and receiver and [math]\displaystyle{ r_{rcv}^{sat} }[/math] is the distance between them. The constants [math]\displaystyle{ c = 299792458 m/s }[/math] and [math]\displaystyle{ c=299792458\,m/s }[/math] and [math]\displaystyle{ \mu=G\,M_\oplus=3.986004418 \cdot 10^{14}\, m^3/s^2 }[/math] are the sped of light and the Earth's gravitational constant, respectively (see Reference Frames in GNSS).
This correction must be added to the Euclidian distance given by the following equation (2).
- [math]\displaystyle{ \rho_{rcv}^{sat}=\left\| {r}_{rcv}-{r}^{sat}\right \|=\sqrt{(x_{rcv}-x^{sat})^2+(y_{rcv}-y^{sat})^2+(z_{rcv}-z^{sat})^2} \qquad \mbox{(2)} }[/math]
Next figure 1 illustrates with an example the Shapiro signal propagation delays for the satellites in view from a receiver in Barcelona, Spain (receiver coordinates [math]\displaystyle{ \lambda\simeq 2^ o $\phi \simeq 41^o }[/math]).
A very good review of relativistic effects on GPS can be found in [Ashby, N., 2003] [1] , (see http://relativity.livingreviews.org/Articles/lrr-2003-1).
References
- ^ [Ashby, N., 2003] Ashby, N., 2003. Relativity in the Global Positioning System. http://relativity.livingreviews.org/articles/lrr-2003-1/.