If you wish to contribute or participate in the discussions about articles you are invited to contact the Editor
Ellipsoidal and Cartesian Coordinates Conversion: Difference between revisions
Carlos.Lopez (talk | contribs) No edit summary |
Jaume.Sanz (talk | contribs) No edit summary |
||
| Line 1: | Line 1: | ||
{{Article Infobox2 | {{Article Infobox2 | ||
|Category=Fundamentals | |Category=Fundamentals | ||
|Authors=J. Sanz Subirana, J.M. Juan Zornoza and M. Hernández-Pajares, Technical University of Catalonia, Spain. | |||
|Authors= J. Sanz Subirana, | |||
|Level=Advanced | |Level=Advanced | ||
|YearOfPublication=2011 | |YearOfPublication=2011 | ||
|Logo=gAGE | |Logo=gAGE | ||
|Title={{PAGENAME}} | |||
}} | }} | ||
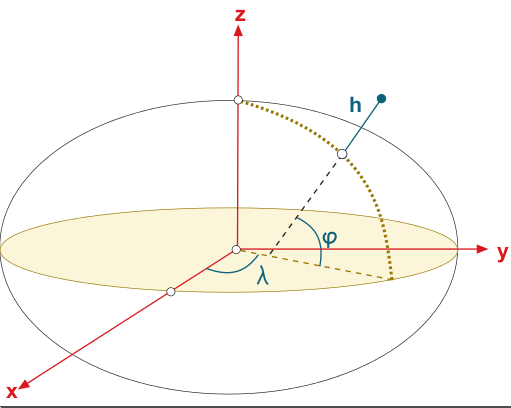
The <math>(x,y,z)</math> ECEF cartesian coordinates can be expressed in the ellipsoidal coordinates <math>(\lambda,\varphi, h)</math>, where <math>\lambda</math> and <math>\varphi</math> are, respectively, the ellipsoidal longitude and latitude, and <math>h</math> the height above the tangent plain to ellipsoid at the point coordinates <math>(x,y,z)</math>. | The <math>(x,y,z)</math> ECEF cartesian coordinates can be expressed in the ellipsoidal coordinates <math>(\lambda,\varphi, h)</math>, where <math>\lambda</math> and <math>\varphi</math> are, respectively, the ellipsoidal longitude and latitude, and <math>h</math> the height above the tangent plain to ellipsoid at the point coordinates <math>(x,y,z)</math>. | ||
Revision as of 10:38, 18 January 2012
| Fundamentals | |
|---|---|
| Title | Ellipsoidal and Cartesian Coordinates Conversion |
| Author(s) | J. Sanz Subirana, J.M. Juan Zornoza and M. Hernández-Pajares, Technical University of Catalonia, Spain. |
| Level | Advanced |
| Year of Publication | 2011 |
The [math]\displaystyle{ (x,y,z) }[/math] ECEF cartesian coordinates can be expressed in the ellipsoidal coordinates [math]\displaystyle{ (\lambda,\varphi, h) }[/math], where [math]\displaystyle{ \lambda }[/math] and [math]\displaystyle{ \varphi }[/math] are, respectively, the ellipsoidal longitude and latitude, and [math]\displaystyle{ h }[/math] the height above the tangent plain to ellipsoid at the point coordinates [math]\displaystyle{ (x,y,z) }[/math].
Figure 1 illustrates the relation between Cartesian and ellipsoidal coordinates.
From ellipsoidal to Cartesian coordinates
The Cartesian coordinates of a point [math]\displaystyle{ (x,y,z) }[/math] can be obtained from the ellipsoidal coordinates [math]\displaystyle{ (\varphi, \lambda, h) }[/math] by the next expressions:
- [math]\displaystyle{ \begin{array}{l} x=(N+h) \cos \varphi\,\cos \lambda\\ y=(N+h)\cos \varphi\,\sin \lambda\\ z=\left ((1-e^2)N+h \right)\sin \varphi\\ \end{array} \qquad \mbox{(1)} }[/math]
where [math]\displaystyle{ N }[/math] is the radius of curvature in the prime vertical:
- [math]\displaystyle{ N=\displaystyle \frac{a}{\sqrt{1-e^2\sin^2\varphi}} \qquad \mbox{(2)} }[/math]
and where the eccentricity [math]\displaystyle{ e }[/math] is related with the semi-major axis [math]\displaystyle{ a }[/math], the semi-minor axis [math]\displaystyle{ b }[/math] and the flattening factor [math]\displaystyle{ f }[/math] by:
- [math]\displaystyle{ e^2=\displaystyle \frac{a^2-b^2}{a^2}=2f-2f^2 \qquad \mbox{(3)} }[/math]
From Cartesian to ellipsoidal coordinates
The ellipsoidal coordinates of a point [math]\displaystyle{ (\varphi, \lambda, h) }[/math] can be obtained from the cartesian coordinates [math]\displaystyle{ (x,y,z) }[/math] as follows:
The longitude [math]\displaystyle{ \lambda }[/math] is given by: [math]\displaystyle{ \lambda= \arctan\frac{y}{x} \qquad \mbox{(4)} }[/math]
The latitude is computed by an iterative procedure.
- 1. The initial value is given by:
- [math]\displaystyle{ \varphi_{(0)}=\arctan\left [ \frac{z}{(1-e^2)\,p} \right ] \qquad \mbox{(5)} }[/math]
- with [math]\displaystyle{ p=\sqrt{x^2+y^2} }[/math].
- 2. Improved values of [math]\displaystyle{ \varphi }[/math], as well as the height [math]\displaystyle{ h }[/math], are computed iterating in the equations:
- [math]\displaystyle{ \begin{array}{l} N_{{(i)}}= \frac{a}{\sqrt{1-e^2\sin^2\varphi_{_{(i-1)}}}}\\[0.6cm] h_{{(i)}}=\frac{p}{\cos \varphi_{_{(i-1)}}}-N_{(i)}\\[0.5cm] \varphi_{{(i)}}= \arctan\left [ \frac{z}{ \left ( 1 - e^2\frac{N_{(i)}}{N_{(i)}+h_{(i)}} \right ) p } \right ] \end{array} \qquad \mbox{(6)} }[/math]
- The iterations are repeated until the change between two successive values of [math]\displaystyle{ \varphi_{(i)} }[/math] are smaller than the precision required.