If you wish to contribute or participate in the discussions about articles you are invited to contact the Editor
WARTK Systems
| Fundamentals | |
|---|---|
| Title | WARTK Systems |
| Author(s) | GMV |
| Level | Basic |
| Year of Publication | 2011 |
The Wide Area RTK (WARTK) concept was introduced in the late 1990s by the Research Group of Astronomy and Geomatics (gAGE) from the Technical University of Catalonia (UPC). The WARTK method dramatically increases the RTK/NRTK service area, with permanent stations separated by up to 500–900 kilometers. RTK reference stations should be of the order of ten km distance from rover, because the ionosphere produces ambiguity estimation biases that lead to positioning error above 1 meter. The main WARTK techniques are related to an accurate real-time computation of ionospheric corrections, combined with an optimal processing of GNSS observables (carrier phases in particular) in both 2 and 3-frequency GNSS systems.
WARTK Systems
User Receiver that can applied WARTK techniques are not still in the market. The gAGE/UPC group have been done several test with simulated data and also with real data. In the case of WARTK-3 the work has been done together with ESTEC/ESA, as simulated data from GALILEO constellation is needed. In the following, the most representative experiments carried with WARTK are described:
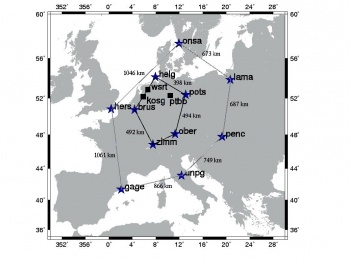
- Test for WARTK-2 technique: Results during the recent Solar Maximum peak, with several European stations (see figure) during four consecutive days, 110-113 of 2000. In this scenario the geomagnetic activity is low to moderate, but the typical vertical Total Electron Content value at noon is 60 TECU (and STEC until 300 TECU and more), i.e. 3 times the values in 1998.
The main network, i.e. the stations used to solve on the fly the ambiguities and to get the real-time Zenith Tropospheric Delay (ZTD), are formed by the IGS permanent receivers BRUS, POTS, OBER and HELG (see figure), and WSRT that will be treated as rover receiver. An additional ring of IGS receivers, HERS, ONSA, LAMA, PENC and UNPG, and the permanent Ashtech Z-XII receiver at gAGE (UPC university), have been used only to compute the ionospheric model. The selection of this data set has been constrained to meet several additional criteria, among those mentioned for Solar Maximum peak: (1) avoiding as many Rogue receivers, and using as many Ashtech receivers as possible (worst and best performance, respectively, in scenarios with severe variations of ionospheric refraction [1]), (2) To have distances of WARTK networks (more than 300 km between the reference stations). Once the ionospheric tomographic model is updated in real-time mode, the double differences of the widelane ambiguities are computed. In this case with extremely high STEC values, it is specially important to incorporate in the real-time tomographic ionospheric model the resolved ambiguities as constrains in ∇ΔSTEC: in this way a success rate of more than 80% at elevations lower than 20 degrees and 90% at 25 degrees are obtained. However, if the fixed ambiguities are not incorporated in the ionospheric model, the success diminishes to about 10% below 50 degrees of elevation. This matters most in the afternoon, when the highest STEC values happen. After fixing, in real-time mode, the full set of ambiguities, the tropospheric refraction obtained agrees with the postprocessed solutions to about 1 cm (or better, in some periods), and with maximum deviations of less than 3 cm. These results are obtained in the context of extreme ionospheric conditions that reduce the number of ambiguities resolved, especially in the afternoon, reducing the amount of useful data to compute the real-time troposphere. In the resolution of the rover receiver ambiguities, the success rate for both ambiguities ∇ΔN1, ∇ΔN2, after fixing on the fly ambiguities, its own resolved ambiguities is typically about 75%, and between 100% and 50% in the afternoon, due to the extreme ionospheric conditions (the success diminish a 25% if the ambiguities of the rover are not asimilated OTF). This affects the resolution of the ZTD in the rover with the present strategy resulting in an RMS with the corresponding postprocess solution of 1.3 cm. The corresponding solution in real-time floating the ambiguities (RTROP) provides very bad results (RMS of 3.6 cm). The comparison with Precise Point Positioning approach provides an RMS of 1.5 cm, in front of 4.2 cm with RTROP.
- Test for WARTK-3 technique[2]: The WARTK technique for 3 frequencies has been proved with simulated data. The ROVE experiment simulated one surface rove receiver navigating in Catalonia. The closest reference receiver used was CREU, at 178 km away, having 6 additional fixed station around (in Spain, Portugal and France). The simulated scenario is under Solar Maximum conditions (worst case ionospheric scenario).
The first point which performance has been analyzed is the error of the real-time ionospheric corrections provided to the user (or interpolation problem). The International Reference Ionosphere model (IRI, Bilitza 1990) has been used to simulate the ionospheric delays. Such model predicts realistic ionospheric refraction values, but without considering ionospheric waves (Traveling Ionospheric Disturbances, TIDs). Moreover, the study concentrates on the ionospheric interpolation problem, by considering that the carrier phase ambiguities between the reference permanent GNSS stations can be fixed correctly in real-time (this has been proven with actual GPS up to baselines of thousands of kilometers in WARTK-2 tests). One important result obtained in this scenario is that with the corresponding high ionospheric values, a planar fit is not accurate enough for the interpolation task. However a 2nd order (quadratic) interpolation procedure of the between-stations single differences is accurate enough to guarantee the achievement of errors below the exigent limit of 2.5 cm of the ionospheric combination, S1-S2=Si. This is due to the high values and variations achieved by the ionospheric refraction in the Solar Maximum scenario and, the sometimes strong ionospheric slant TEC variation of the rays coming from the South and crossing tails of the Equatorial Anomalies. Indeed, in this scenario, the user ionospheric interpolation error decreases when we pass from using planar fit (below 7 cm) to using quadratic interpolation (below 2 cm). In this way, with the quadratic interpolation, 100% of real-time interpolated double-differenced STEC at ROVE are below the threshold value of 2.5 cm of Si. Then, the user will apply the ionospheric corrections received from the fixed stations network in the framework of a general navigation Kalman filter feed with zero-differenced (undifferenced) observations. With 3-frequencies measurements 100% of ambiguities are fixed since the beginning and the real-time positioning error decrease below 10 cm after a convergence time of just few seconds, needed to decorrelate the tropospheric delay. The results are practically equivalent in single epoch mode (with an RMS of 1.4 cm) emulated as a continuous cold start (setting up all the variances to ∞), but maintaining the random walk tropospheric estimation. Comparing the corresponding performance with 2-frequencies systems, when simulating a cold starting-up, a sub-decimeter real-time positioning is obtained such as with three-frequencies (RMS of 2 cm and 100% amb. fixed), but after a convergence time of approximately 100 sec (time needed for both ambiguity fixing and initial tropospheric state estimation), instead of instantaneously. This result is in concordance with that obtained with actual GPS data. The corresponding positioning errors for ROVE moving around the scenario are lower than 6 cm, obtaining a RMS of 2.4 cm (3-D), with an RMS of 1.7, 0.4 and 1.5 cm in X,Y,Z components, respectively.
Notes