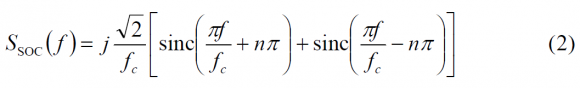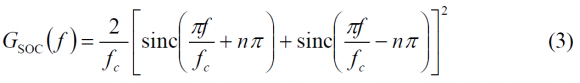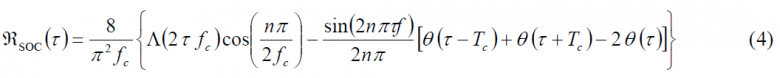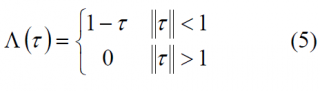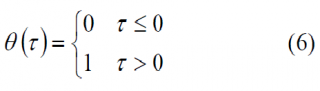If you wish to contribute or participate in the discussions about articles you are invited to contact the Editor

Sinusoidal Binary Offset Carrier (SOC) Signals: Difference between revisions

From Navipedia
Jump to navigation Jump to search
(Created page with "{{Article Infobox2 |Category=Fundamentals |Title={{PAGENAME}} |Authors= J.A Ávila Rodríguez, University FAF Munich, Germany. |Level=Advanced |YearOfPublication=2011 }} To deriv...")
 
No edit summary
 
Line 1: Line 1:
{{Article Infobox2
{{Article Infobox2
|Category=Fundamentals
|Category=Fundamentals
|Title={{PAGENAME}}
|Authors=J.A Ávila Rodríguez, University FAF Munich, Germany.
|Authors= J.A Ávila Rodríguez, University FAF Munich, Germany.
|Level=Advanced
|Level=Advanced
|YearOfPublication=2011
|YearOfPublication=2011
|Title={{PAGENAME}}
}}
}}
To derive the spectrum of the SOC signals, the most convenient is to use the convolution theorem. According to it, the Fourier transform of the chip waveform can be expressed in terms of a convolution between the modulating carrier and the code bit. The problem reduces then to calculating the Fourier transforms for each signal as shown in [J. Winkel, 2002] <ref name= "WIK_2002"> [J. Winkel, 2002] J. Winkel, Modeling and Simulating Generic GNSS Signal Structures and Receiver in a Multipath Environment, Ph.D. Thesis, University FAF Munich, Neubiberg, Germany.</ref>. In fact:
To derive the spectrum of the SOC signals, the most convenient is to use the convolution theorem. According to it, the Fourier transform of the chip waveform can be expressed in terms of a convolution between the modulating carrier and the code bit. The problem reduces then to calculating the Fourier transforms for each signal as shown in [J. Winkel, 2002] <ref name= "WIK_2002"> [J. Winkel, 2002] J. Winkel, Modeling and Simulating Generic GNSS Signal Structures and Receiver in a Multipath Environment, Ph.D. Thesis, University FAF Munich, Neubiberg, Germany.</ref>. In fact:
Line 39: Line 39:
==References==
==References==
<references/>
<references/>
== Credits ==
The information presented in this NAVIPEDIA’s article is an extract of the PhD work performed by Dr. Jose Ángel Ávila Rodríguez in the FAF University of Munich as part of his Doctoral Thesis “On Generalized Signal Waveforms for Satellite Navigation” presented in June 2008, Munich (Germany)




[[Category:Fundamentals]]
[[Category:Fundamentals]]
[[Category:GNSS Signals]]
[[Category:GNSS Signals]]

Latest revision as of 09:18, 25 November 2011


FundamentalsFundamentals
Title Sinusoidal Binary Offset Carrier (SOC) Signals
Author(s) J.A Ávila Rodríguez, University FAF Munich, Germany.
Level Advanced
Year of Publication 2011

To derive the spectrum of the SOC signals, the most convenient is to use the convolution theorem. According to it, the Fourier transform of the chip waveform can be expressed in terms of a convolution between the modulating carrier and the code bit. The problem reduces then to calculating the Fourier transforms for each signal as shown in [J. Winkel, 2002] [1]. In fact:

SOC Eq 1.png


which can be further simplified as follows, assuming that the code is ideal:

SOC Eq 2.png


Therefore, the power spectral density adopts the following form:

SOC Eq 3.png


It is interesting to note also that the same distinction between even and odd SOCs can also be made here as with the rectangular signals that we have already studied. Furthermore, the maximum of the spectrum is not located at [math]\displaystyle{ f = n f_c }[/math] as one might expect, but somewhere close to that point as shown in [J. Winkel, 2002] [1]. Finally, the autocorrelation function of the SOC signal for the sine-phased case is shown to be [J. Winkel, 2002] [1]:

SOC Eq 4.png


where [math]\displaystyle{ \Lambda \left ( \tau \right ) }[/math] is the triangular function and [math]\displaystyle{ \Theta \left ( \tau \right ) }[/math] represents the Heaviside step function. As we know the triangular function is defined as follows:

SOC Eq 5.png


and the Heaviside step function is equally shown to be defined as:

SOC Eq 6.png


References

  1. ^ a b c [J. Winkel, 2002] J. Winkel, Modeling and Simulating Generic GNSS Signal Structures and Receiver in a Multipath Environment, Ph.D. Thesis, University FAF Munich, Neubiberg, Germany.


Credits

The information presented in this NAVIPEDIA’s article is an extract of the PhD work performed by Dr. Jose Ángel Ávila Rodríguez in the FAF University of Munich as part of his Doctoral Thesis “On Generalized Signal Waveforms for Satellite Navigation” presented in June 2008, Munich (Germany)

Retrieved from "https://gssc.esa.int/navipedia/index.php?title=Sinusoidal_Binary_Offset_Carrier_(SOC)_Signals&oldid=10148"