Solid Tides

Fundamentals
Title	Solid Tides
Author(s)	J. Sanz Subirana, JM. Juan Zornoza and M. Hernandez-Pajares, University of Catalunia, Spain.
Level	Advanced
Year of Publication	2011

Solid tides comprise the Earth's crust movement (and thus the receiver location coordinates variation) due to gravitational attracting forces produced by external bodies, mainly the sun and the moon. The Solid Tides produce vertical and horizontal displacements that can be expressed by spherical harmonics expansion [math]\displaystyle{ (m,n) }[/math], characterised by the Love and Shida numbers [math]\displaystyle{ h_{mn} }[/math], [math]\displaystyle{ l_{mn} }[/math].

A simplified model for the tide displacement, but with few millimetres of accuracy, is given by the following expression (from IERS Conventions degree 2 tides displacement model --in-phase corrections--, see [Denis et al., 2004] , page 79):

[math]\displaystyle{ \Delta {\mathbf r}= \sum_{j=2}^{3}{\frac{G\,M_j\,R_e^4}{G\,M_\oplus\,R_j^3}} \left \{h_2 \,\hat{\mathbf r} \left ( \frac{3}{2} (\hat{\mathbf R}_j \cdot \hat{\mathbf r})^2 -\frac{1}{2}\right) + 3\,l_2\,(\hat{\mathbf R}_j \cdot \hat{\mathbf r}) \left [\hat{\mathbf R}_j-(\hat{\mathbf R}_j \cdot \hat{\mathbf r})\,\hat{\mathbf r} \right ]\right \} \qquad\mbox{(1)} }[/math]

[math]\displaystyle{ \Delta {\mathbf r} }[/math] is a site displacement vector in a Cartesian coordinates system.

[math]\displaystyle{ G\,M_\oplus }[/math] is gravitational parameters of the earth.

[math]\displaystyle{ G\,M_j }[/math] is gravitational parameters of the moon ([math]\displaystyle{ j = 2 }[/math]) and sun ([math]\displaystyle{ j = 3 }[/math]).

([math]\displaystyle{ M_{sun}/M_\oplus=332946.0 }[/math], [math]\displaystyle{ M_{moon}/M_\oplus=0.01230002 }[/math])

[math]\displaystyle{ \hat{\mathbf R}_j }[/math], [math]\displaystyle{ \displaystyle R_j }[/math] are the unit vector from the geocentre to moon or sun and the magnitude of that vector.

[math]\displaystyle{ R_e }[/math] is the earth's equatorial radius ([math]\displaystyle{ R_e=6378136.6\,m }[/math]).

[math]\displaystyle{ \hat{\mathbf r} }[/math], [math]\displaystyle{ r }[/math] are the unit vector from the geocentre to the station and the magnitude of that vector.

[math]\displaystyle{ h_2 }[/math] & is the nominal degree 2 Love number ([math]\displaystyle{ h_2=0.6078 }[/math]).

[math]\displaystyle{ l_2 }[/math] & is the nominal degree 2 Shida number ([math]\displaystyle{ l_2=0.0847 }[/math]).

Notice that the radial (not vertical) component ([math]\displaystyle{ \hat{\mathbf r} }[/math]) is proportional to the Love number [math]\displaystyle{ h_2 }[/math], while the terms in [math]\displaystyle{ l_2 }[/math] corresponds to components orthogonal to the radial direction (not the horizontal plane).

A small correction of latitude ([math]\displaystyle{ \varphi }[/math]) dependence can be considered in the [math]\displaystyle{ h_2 }[/math] and [math]\displaystyle{ l_2 }[/math] values of previous equation:

[math]\displaystyle{ N=nint\left[\frac{\Delta \Phi_{previo}-\delta \Phi}{2\pi}\right] \qquad \zeta=\hat{k}\cdot \left(\vec{D}^{\prime}\times\vec{D}\right) }[/math]

according to the expression:

[math]\displaystyle{ \begin{array}{rl} h_2&= 0.6078-0.0006\left [(3 \sin^2\varphi-1)/2\right]\\[0.3cm] l_2&= 0.0847+0.0002\left [(3 \sin^2\varphi-1)/2\right] \end{array} \qquad\mbox{(2)} }[/math]

An additional refinement can be to take into account the additional contribution due to the degree 3 tides [Denis et al., 2004], page 80):

[math]\displaystyle{ \Delta {\mathbf r}= \sum_{j=2}^{3}{\frac{G\,M_j\,R_e^5}{G\,M_\oplus\,R_j^4}} \left \{h_3 \,\hat{\mathbf r} \left ( \frac{5}{2} (\hat{\mathbf R}_j \cdot \hat{\mathbf r})^3 -\frac{3}{2} (\hat{\mathbf R}_j \cdot \hat{\mathbf r})\right) + l_3\,\left (\frac{15}{2} (\hat{\mathbf R}_j \cdot \hat{\mathbf r})^2 - \frac{3}{2} \right ) \left [\hat{\mathbf R}_j-(\hat{\mathbf R}_j \cdot \hat{\mathbf r})\,\hat{\mathbf r} \right ]\right \} \qquad\mbox{(2)} }[/math]

where [math]\displaystyle{ h_3=0.292 }[/math] and [math]\displaystyle{ l_3=0.015 }[/math]. Only the moon's contribution ([math]\displaystyle{ j=2 }[/math]) need to be computed, because the contribution of sun ([math]\displaystyle{ j=3 }[/math]) is negligible. Nevertheless, also the moon's contribution to this degree 3 tide to the radial displacement does not exceed the [math]\displaystyle{ 1.7 }[/math] millimetres in radial and [math]\displaystyle{ 0.02 }[/math] millimetres in transversal components.

Finally, it must be taken into account that the previous equations provide the correction to obtain the coordinates relative to the "conventional tide free". To obtain the position relative to the "mean tide" the following vector must be added :

[math]\displaystyle{ \begin{array}{ll} \left [-0.1206+0.0001\,P_2(\sin \varphi)\right ]P_2(\sin \varphi) \qquad\mbox{(m) radial direction}\\ \left [-0.0252+0.0001\,P_2(\sin \varphi)\right ]\sin 2\varphi \qquad\mbox{(m) north direction} \end{array} \qquad\mbox{(3)} }[/math]

where [math]\displaystyle{ P_2(\sin \varphi)=(3\,\sin^2\varphi-1)/2 }[/math].

Notice that the radial component of this part can amount for about [math]\displaystyle{ -12 }[/math] centimetres at the poles and about [math]\displaystyle{ +6 }[/math] centimetres at the equator.

An alternative set of equations describing a similar model implemented in GIPSY-OASIS II can be found in [Webb and Zumberge, 1993]

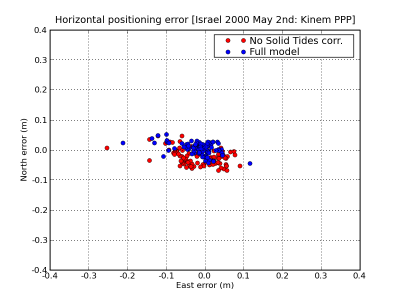
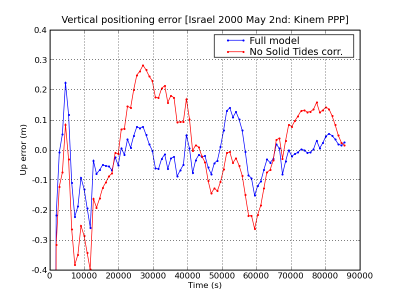
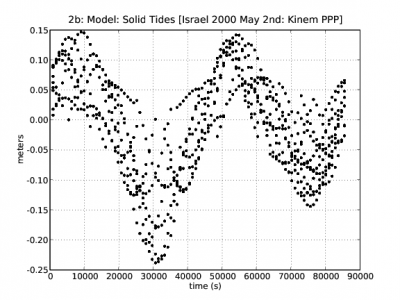
Figure 1 illustrates the effect of the Solid Tides correction. The navigation solution computed using the solid tides correction is shown in blue and the solution without using this correction in red. The effect of solid tides displacement on range is shown in the second row at left.

Figure 1: Solid Tides: Range and position domain effect

frameless	frameless
frameless	First row shows the horizontal (left) and vertical (right) positioning error using (blue) or not using (red) the solid tides correction (from equations (1) to (3). The effect on range of solid tides displacement is shown in the second row at left.