If you wish to contribute or participate in the discussions about articles you are invited to contact the Editor

Sidereal Time: Difference between revisions

From Navipedia
Jump to navigation Jump to search
No edit summary
No edit summary
 
(13 intermediate revisions by 2 users not shown)
Line 1: Line 1:
{{Article Infobox2
{{Article Infobox2
|Category=Fundamentals
|Category=Fundamentals
|Authors=J. Sanz Subirana, J.M. Juan Zornoza and M. Hernández-Pajares, Technical University of Catalonia, Spain.
|Level=Basic
|YearOfPublication=2011
|Title={{PAGENAME}}
|Title={{PAGENAME}}
|Authors= J. Sanz Subirana, JM. Juan Zornoza and M. Hernandez-Pajares, University of Catalunia, Spain.
|Level=Medium
|YearOfPublication=2011
|Logo=gAGE
}}
}}
 
The reference is the Vernal Equinox, or the Aries point, which is defined as the intersection between the equator and the ecliptic plane <ref group="footnotes"> Ecliptic: Apparent circular path of the sun on the celestial sphere during the course of a year. The plane of the ecliptic is inclined an angle of about 23<math>^{o}</math>26<math>^{'}</math> with respect to the celestial equator, see equation (5) in [[ICRF to CEP]].</ref>. Two types of Aries point can be considered depending on which equator plane is considered (i.e, the mean equator or the true equator).
The reference I s the Vernal Equinox, or the Aries point, which is defined as the intersection between the equator and the ecliptic plane <ref group="footnotes"> Ecliptic: Apparent circular path of the sun on the celestial sphere during the course of a year. The plane of the ecliptic is inclined an angle of about 23<math>^{o}</math>26<math>^{\prime}</math> with respect to the celestial equator.</ref>. Two types of Aries point can be considered depending on which equator plane is considered (i.e, the mean equator or the true equator).




*'' Mean Aries point'': Is the intersection of mean equator (the effect of Precession over the earth rotation axis is taken into account, see article [[Transformation between Celestial and Terrestrial Frames]]) with ecliptic <ref group="footnotes">Actually, the mean ecliptic.  Like earth rotation pole, Ecliptic pole suffers a Precession and Nutation effect due to the perturbation of moon and major planets on earth orbit. Nevertheless its amplitude is 50 times shorter than the earth rotation pole and, at the level of accuracy required here, we will not distinguish between mean or true ecliptic.</ref>.
*'' Mean Aries point'': Is the intersection of mean equator (the effect of Precession over the earth rotation axis is taken into account, see article [[Transformation between Celestial and Terrestrial Frames]]) with ecliptic <ref group="footnotes">Actually, the mean ecliptic.  Like earth rotation pole, Ecliptic pole suffers a Precession and Nutation effect due to the perturbation of moon and major planets on earth orbit. Nevertheless its amplitude is 50 times shorter than the earth rotation pole and, at the level of accuracy required here, we will not distinguish between mean or true ecliptic.</ref>, see figure 1.




Line 23: Line 21:
According to the previous definitions, four classes of sidereal times are introduced:
According to the previous definitions, four classes of sidereal times are introduced:


* ''LAST or Local "Apparent" Sidereal Time'' (<math>\Theta</math>): is the hour angle of  the "true" Aries point  (from local meridian) <ref group="footnotes"> Apparent in Astronomy refers to what is seen from an Ideal earth's centre, without atmosphere and rotation. With these conditions the effects of light refraction, light aberration and parallax has been suppressed.</ref>.
* ''Local "Apparent" Sidereal Time'' (LAST)(<math>\Theta</math>): is the hour angle of  the "true" Aries point  (from local meridian) <ref group="footnotes"> Apparent in Astronomy refers to what is seen from an Ideal earth's centre, without atmosphere and rotation. With these conditions the effects of light refraction, light aberration and parallax has been suppressed.</ref>.


* ''GAST or Greenwich "Apparent" Sidereal Time'' (<math>\Theta_{_G}</math>): is the hour angle of  "true" Aries point, from Greenwich meridian.
* ''Greenwich "Apparent" Sidereal Time'' (GAST)(<math>\Theta_{_G}</math>): is the hour angle of  "true" Aries point, from Greenwich meridian.


*'' LMST or Local Mean Sidereal Time'' (<math>\theta</math>): the same that LAST with the mean equinox.
*''Local Mean Sidereal Time'' (LMST)(<math>\theta</math>): the same that LAST with the mean equinox.


* ''GMST or Greenwich Mean Sidereal Time'' (<math>\theta_{_G}</math>): the same that GAST with the mean equinox.
*''Greenwich Mean Sidereal Time'' (GMST)(<math>\theta_{_G}</math>): the same that GAST with the mean equinox.




The Figure 2 summaries these four sidereal times.
The Figure 2 summaries these four sidereal times.


::[[File: Siderial_Time_Fig_2.png|none|thumb|480px|'''''Figure 2:''''' Different sidereal Times (from > [Seeber, 1993]  <ref> [Seeber, 1993] Seeber, G., 1993. Satellite Geodesy: Foundations, Methods, and Applications. Walter de Gruyter & Co., Berlin, Germany.</ref>]]
::[[File: Siderial_Time_Fig_2.png|none|thumb|480px|'''''Figure 2:''''' Different sidereal Times (from [Seeber, 1993]) <ref> [Seeber, 1993] Seeber, G., 1993. Satellite Geodesy: Foundations, Methods, and Applications. Walter de Gruyter & Co., Berlin, Germany.</ref>]]
 
GAST and GMST are given by equations (3) and (4) in [[CEP to ITRF]], respectively. Local and Greenwich Sidereal Times differ by the longitude <math>\displaystyle \lambda</math> of the local meridian. The difference between Apparent and Mean Sidereal Times is called ''Equation of Equinoxes'' (where <math>\displaystyle \alpha_E</math> is given by equation (7) in [[CEP to ITRF]]):


::<math>
\begin{array}{lcl}
GMST\,-\,LMST\,=\,GAST\,-\,LAST\,=\,\lambda \\
GMST\,-\,GAST\,=\,LMST\,-\,LAST\,=\,\alpha_E
\end{array}
\qquad \mbox{(1)}
</math>


==Notes==
==Notes==

Latest revision as of 11:41, 23 February 2012


FundamentalsFundamentals
Title Sidereal Time
Author(s) J. Sanz Subirana, J.M. Juan Zornoza and M. Hernández-Pajares, Technical University of Catalonia, Spain.
Level Basic
Year of Publication 2011

The reference is the Vernal Equinox, or the Aries point, which is defined as the intersection between the equator and the ecliptic plane [footnotes 1]. Two types of Aries point can be considered depending on which equator plane is considered (i.e, the mean equator or the true equator).




Figure 1: Mean and True equinox.


According to the previous definitions, four classes of sidereal times are introduced:

  • Local "Apparent" Sidereal Time (LAST)([math]\displaystyle{ \Theta }[/math]): is the hour angle of the "true" Aries point (from local meridian) [footnotes 5].
  • Greenwich "Apparent" Sidereal Time (GAST)([math]\displaystyle{ \Theta_{_G} }[/math]): is the hour angle of "true" Aries point, from Greenwich meridian.
  • Local Mean Sidereal Time (LMST)([math]\displaystyle{ \theta }[/math]): the same that LAST with the mean equinox.
  • Greenwich Mean Sidereal Time (GMST)([math]\displaystyle{ \theta_{_G} }[/math]): the same that GAST with the mean equinox.


The Figure 2 summaries these four sidereal times.

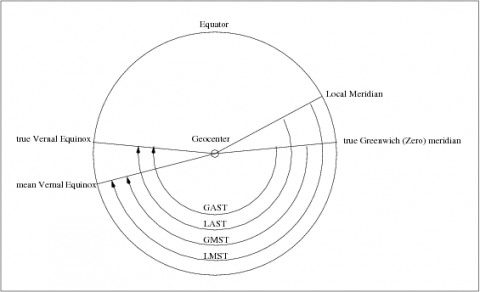Figure 2: Different sidereal Times (from [Seeber, 1993]) [1]

GAST and GMST are given by equations (3) and (4) in CEP to ITRF, respectively. Local and Greenwich Sidereal Times differ by the longitude [math]\displaystyle{ \displaystyle \lambda }[/math] of the local meridian. The difference between Apparent and Mean Sidereal Times is called Equation of Equinoxes (where [math]\displaystyle{ \displaystyle \alpha_E }[/math] is given by equation (7) in CEP to ITRF):

[math]\displaystyle{ \begin{array}{lcl} GMST\,-\,LMST\,=\,GAST\,-\,LAST\,=\,\lambda \\ GMST\,-\,GAST\,=\,LMST\,-\,LAST\,=\,\alpha_E \end{array} \qquad \mbox{(1)} }[/math]

Notes

  1. ^ Ecliptic: Apparent circular path of the sun on the celestial sphere during the course of a year. The plane of the ecliptic is inclined an angle of about 23[math]\displaystyle{ ^{o} }[/math]26[math]\displaystyle{ ^{'} }[/math] with respect to the celestial equator, see equation (5) in ICRF to CEP.
  2. ^ Actually, the mean ecliptic. Like earth rotation pole, Ecliptic pole suffers a Precession and Nutation effect due to the perturbation of moon and major planets on earth orbit. Nevertheless its amplitude is 50 times shorter than the earth rotation pole and, at the level of accuracy required here, we will not distinguish between mean or true ecliptic.
  3. ^ This equator is defined as the plane that contains the Geocenter and is orthogonal to the instantaneous daily rotation axis.
  4. ^ Due to the accuracies needed, it is enough to compute the true Aries point using mean Ecliptic plane. We will refer to this plane always as "the ecliptic", without distinguishing between the mean or the true one.
  5. ^ Apparent in Astronomy refers to what is seen from an Ideal earth's centre, without atmosphere and rotation. With these conditions the effects of light refraction, light aberration and parallax has been suppressed.

References

  1. ^ [Seeber, 1993] Seeber, G., 1993. Satellite Geodesy: Foundations, Methods, and Applications. Walter de Gruyter & Co., Berlin, Germany.
Retrieved from "https://gssc.esa.int/navipedia/index.php?title=Sidereal_Time&oldid=11651"