If you wish to contribute or participate in the discussions about articles you are invited to contact the Editor
Earth Sciences
| Applications | |
|---|---|
| Title | Earth Sciences |
| Author(s) | ESA |
| Edited by | GMV |
| Level | Intermediate |
| Year of Publication | 2013 |
Earth Science covers sciences related to understanding the planet Earth, being the use of GNSS signals most relevant on the aspects of Earth physics (as opposed to chemistry or biology). The main fields of this science that are studied using GNSS as tools are:
- Geodesy and Geodynamics;
- Remote Sensing Using GNSS-R;
- Physics of the Ionosphere and Troposphere.
Geodesy and Geodynamics
The International Association of Geodesy (IAG) organized the Global Geodetic Observing System (GGOS) composed by several IAG technique-services that aims mainly the support of the Earth Science research. The service that provides the means to use GNSS data is the International GNSS Service (IGS) that nowadays has a fundamental contribution to the overall GNSS community since provides the highest quality data and products as the standard for Global Navigation Satellite Systems (GNSS). In addition there are also services combining the products of the technique specific services, such as the International Earth Rotation and Reference Systems Service (IERS). The GGOS enables research in three fundamental areas of geodesy:
- The geometric shape of the Earth (land, ice and ocean surface) as well as its variation in time;
- The orientation of the Earth in inertial space as a function of time;
- The Earth’s gravity field and its temporal variations.
Introduction
One of the main information sources of satellite geodesy is provided by the dynamics of satellite orbits. Satellite motion is conveniently described in a geocentric but otherwise inertial coordinate system that may be called quasi-inertial and is of the main Reference Systems and Frames used in satellite navigation. As the gravitational attraction of a non-spherical Earth is the dominant constituent of the force field acting on the satellite, the Earth’s orientation in the quasi-inertial system must be known as a function of time in order to generate the ephemerides of a satellite. The Earth’s rotation is described by the three Euler angles (namely precession and nutation in longitude and latitude, and Universal Time (UT)). The quantities related to the first time derivatives of the three Euler angles can be established accurately by satellite-geodetic methods. Thus, GNSS-based nutation rates are valuable contributions to determine high frequency terms of the nutation model that are usually retrieved from VLBI observations. Furthermore the Earth Rotation Parameters (ERP), namely the coordinates x and y of polar motion in an Earth-fixed coordinate system and Length of Day (LoD) are also determined by GNSS with unique accuracy.
Measurement of Tectonic Motions and Local Deformations
GNSS have brought greater precision in position determination and above all enabled sustained monitoring at an acceptable cost and in a very efficient and timely way, which enables monitoring variations of geodetic station positioning induced by various geophysical processes. The global network of IGS stations can provide continuous information about motions of the continents or smaller tectonic plates. In addition at a local or regional scale, the ease with which a GNSS can be used and combined with positioning precision using Differential GNSS (DGNSS) techniques or Precise Point Positioning (PPP) technique means that the number of measurement points in areas of seismic or volcanic activity can be increased to monitor changes that might warn of coming disasters. After an earthquake or volcanic eruption, the extent of deformation can be measured too. The International Terrestrial Reference Frame (ITRF) networks (using GNSS data as well other geodetic data) also provides a means of aligning local deformation aiding the attempt to understand the forces behind it on a worldwide scale. In regions of significant seismic activity (e.g. California, Japan), local GNSS networks are used for permanent monitoring of the ground deformations that provides data used to develop or to validate models of crustal movements and earthquakes prediction. In the research of Tectonic Motions and Local Deformations the GNSS contribution is the most substantial and the most productive in combination with other space geodesy techniques[1].
Earth’s Gravity Field and Ocean Surface Determination
One of the main inputs for the ocean surface determination is the altimetry of sufficiently low earth orbit (LEO) satellites accurately measuring the distance between the satellite and the ocean surface. However the orbits are heavily perturbed by the gravity field, by drag, and by radiation pressure and therefore it is of high importance to achieve centimeter precision in the radial direction of the orbits. Precision of this kind can only be achieved by using various space techniques. One of the fundamental contributions to those techniques is the use of onboard GNSS receivers which in combination with GNSS orbits and clock corrections issued by the [1], can provide the position of the LEO satellites as a function of time with centimeter accuracy. Furthermore the use of GNSS for tracking LEOs is a significant improvement in orbit determination of the LEO satellites due continuity and homogeneity. Using GNSS means enables a permanent tracking, 24 hours per day without interruptions. Such coverage could never be achieved with ground tracking systems, where weather conditions (for laser) or visibility limitations (due to the sparseness of the observatories) produce long data gaps.
Atmospheric Waves Generated by Earthquakes and Tsunamis
Acoustic waves excited at the ground, provoked for example by severe weather fronts, nuclear explosions, volcano eruptions, and earthquakes need approximately 10 minutes to reach the F-layer of the ionosphere, where close coupling between the neutral atmosphere and ionized plasma may result in a wavelike modulation of the electron density. Since dual-frequency measurements of navigation signals from GNSS are very sensitive to small plasma changes along the ray path, and therefore earthquake signatures may be detected. The sensitivity of GPS-based ionospheric measurements to earthquakes was first reported by Calais and Minster in 1996[2] by analyzing the extremely strong Denali Park Alaska Earthquake of 3 November 2002. A more detailed analysis of this magnitude 7.9 earthquake has provided objective evidence that seismic-wave-induced upward propagating atmospheric acoustic waves significantly modify the ionospheric plasma measurable by Differential GNSS (DGNSS) phases[3][4]. Whereas earthquakes may excite acoustic waves, tsunamis induce atmospheric gravity waves, which can reach the ionosphere where they may be detected[5]. The time required to manifest a detectable tsunamigenic signature in GNSS-based ionospheric observations may be as short as 20 to 30 minutes but further research is required to confirm this[6][7]. All of these GNSS based techniques are an important contribution for the Earthquakes and Tsunamis research and prediction.
Remote Sensing Using GNSS-R
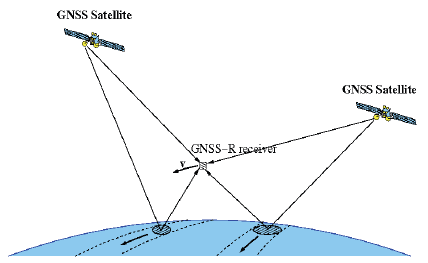
The concept of GNSS-R (GNSS-Reflectometry) or PARIS (Passive Reflectometry and Interferometry System) is that of a bi-static radar, where the transmitter is a GNSS satellite and where the receiver can receive both the signal coming directly from the source and the signal reflected from the Earth’s surface. In spite of the fact that the properties of GNSS signals have been optimized for navigation applications, the reflected signal contains information about the state of the reflecting surface. An illustration of such concept is presented in the figure on the right.
The concept GNSS-R was proposed in 1993 by ESA[8] to provide additional measurements of the sea surface to increase the spatial and temporal resolution provided by radar altimeters. In 1998, the application to remote sensing of the sea roughness and winds was demonstrated[9]. Most of the applications are based on a bi-static radar model adapted to the GNSS-R, as described in[10].
When the signals are reflected at grazing angles, as is the case in the lower parts of the GNSS radio occultation, they maintain coherence and interfere with the direct signal. This is a common situation, observable in a large number of GNSS radio occultation events using space GNSS receivers in open loop mode. While these reflected signals contain useful geophysical information[11][12], the term GNSS-R is usually reserved for the non-grazing reflections were the reflected signal is essentially incoherent.
Ocean Surface Altimetry
One of the first applications conceived for GNSS-R was altimetry – the measurement of the ocean surface height and topography. Conventional satellite radar altimeters typically only measure the ocean height at the sub-satellite point (using a nadir-looking real-aperture radar) while the GNSS-R altimeter measures the ocean height at many points at the same time, namely the specular reflection points of all GNSS satellites which are visible above a predefined masking angle. This passive wide-swath altimeter concept lends itself to new applications, such as the detection of tsunamis, ocean eddies or other mesoscale features in ocean height[13][14].
Monitoring Sea State
Since the brightness temperature measured by L-Band radiometers is affected by the sea roughness, the GNSS-R measurements could be utilized to reduce the uncertainties of the sea surface temperature and salinity retrievals. For static coastal platforms an estimation of the sea-state can be obtained by analyzing the interferometric complex field coherence time [15].
Remote Observation of Soil Moisture
The power level of the reflected signal, appropriately normalized, contains information about the soil moisture of the reflecting surface. Thus, GNSS-R data collected from aircraft and/or spacecraft can detect different soil types; urban areas, dry-soil, wetlands[16].
Sea Ice and Dry Snow
A theoretical model to interpret the GNSS signals after reflection by the cryosphere in terms of the firn pack characteristics, accumulation rates and snow roughness and structure is provided in[17]. Promising results obtained in field experiments from aircraft[18][19] and from space[20] have been published.
TEC Monitoring over the Ocean
Another interesting application of GNSS-R is the global mapping of the ionospheric Total Electron Content (TEC) over the ocean. While ground-based GNSS receivers provide good maps of TEC over the populated land masses, there are virtually no observations done over the oceans (apart from the ionospheric profiling done by occultation missions) – leading to severe gaps in the TEC maps which are needed to better understand the spatial and temporal variability of the ionosphere. The GNSS-R altimeter concept described above utilizes at least two frequencies in order to remove the ionospheric delay from the altimetry solution. This delay can be used to reconstruct slant TEC values, one for the link between the GNSS satellite and the LEO platform and one for GNSS-to-ground-to-LEO link in which the two contributions from the ionosphere below the height of the LEO orbit are present. This technique can be suitably combined with a tomographic retrieval algorithm[21].
Physics of the Ionosphere and Troposphere
The carriers and signals as recorded by receivers on/ or near the Earth’s surface have to cross the Earth’s atmosphere, causing signal delay. These delays, namely Ionospheric Delay and Tropospheric Delay, have to be compensated for through measurements or by models in order to achieve the high precision (e.g. orbits and clocks) and availability that it is expected from the system. As a consequence the derived models constitute a valuable input in the study of Earth’s atmosphere behavior as well its effects on radio signals. On the other hand the ionosphere’s effects are frequency dependent and since GNSS satellites transmit signals in more than one frequency this effect can be quite minimized also improving ionospheric analysis.
Ionospheric Monitoring
Ionospheric tomography based on GPS data has been successfully used in regional and wide area Differential GNSS (DGNSS) to improve the location precision and to allow greater distances between the rover and the reference stations. In those cases, ionospheric tomography contributes to the improvement in the resolution of carrier-phase ambiguity. Ionospheric tomography can provide fast and accurate estimates of TEC under conditions of high electron density variability, such as those observed close to the geomagnetic equator, during solar maximum[22]. Tomography modelling is based on a set of three-dimensional cells that covers spatially the sampled ionosphere. In these cells, the electron density is considered constant at a given time. Despite other possibilities to choose the cell distribution, a regular distribution is adequate for describing a region with samples from a more or less homogeneously distributed network of reference stations. The estimate of ionospheric electron density in each cell is based on the ionospheric data obtained from the satellite receiver ray paths crossing that particular cell. Another technique commonly used in the ionospheric monitoring uses the GNSS-R concept above described. Since the space-based receiver on board a LEO satellite is mainly used for accurate positioning and precise timing, the dual frequency navigation measurements can be used to get information on the topside ionosphere above the LEO satellite orbit too. The data enable reconstructing the 3D electron density distribution of the topside ionosphere/plasmasphere in the vicinity of the LEO orbit plane[23]. The more data links from GNSS satellites that are available, the more accurate and higher resolved is the tomographic or assimilative reconstruction of the electron density distribution.
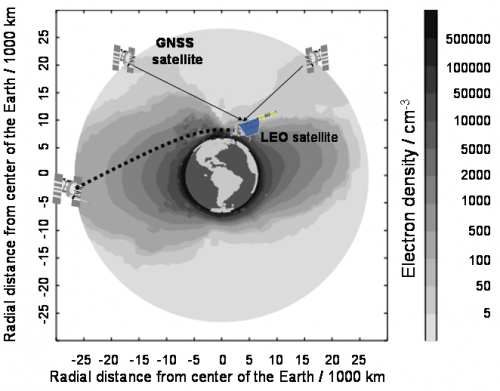
Figure 3 shows the geometry of combined limb-sounding and topside-sounding geometries for reconstruction of the topside ionosphere as it is shown in the background figure based on CHAMP data[24].
Whereas the ground-based measurements are especially suited for imaging the horizontal distribution of plasma, the space-borne measurements are suited to map the vertical distribution of the ionospheric plasma.
Troposphere Monitoring
Microwave signals of the GNSS satellites are time delayed when passing through the atmosphere. Based on this, signal delay parameters, such as the humidity distribution within the troposphere, can be determined. It has already been shown by several international and national projects that the delivery of Zenith Wet Delays (ZWD) derived from a GNSS-network solution with hourly resolution and an accuracy of 1mm in Precipitable Water Vapour (PWV) is achievable. These ZWD-estimates have been successfully assimilated into meteorological models[25]. Preconditions to derive accurate ZWD estimates are accurate satellite orbit and clock information and an almost complete and successful fixing of ambiguities of the chosen phase linear combination. The optimal signal linear combination in terms of wavelength and noise level depends on the baseline length. Typical baseline lengths within Real Time Kinematics (RTK) GNSS-networks which are frequently used for ground-based tropospheric monitoring are between 50 km and 120 km. The set of new signals provided by Galileo as well as GPS/GLONASS ongoing modernization programs will allow for improved ambiguity resolution techniques over such long baselines. Furthermore, GNSS signals at three frequencies will allow modelling of the higher order terms of the ionospheric refraction (on the order of 1 cm), which in turn reduces the error of the tropospheric wet delay estimates.
An example of an application is to use integrated water vapour to monitor climate.
Retrieval of Total Water Vapour Content
Water vapour is an important constituent of the atmosphere, contributing strongly to the weather and playing a critical role in the global climate system. It absorbs and re-radiates energy from the sun and it influences the formation of clouds. Despite its importance to atmospheric processes over a wide range of spatial and temporal scales, it is one of the least understood and poorly described components of the Earth’s atmosphere. Furthermore, it is the most abundant of the greenhouse gases and a better understanding of its role in climate change is needed. Total tropospheric water vapour content can be measured directly by means of a radiosonde (a small meteorological sensor attached to a balloon) or by ground-based microwave radiometry. The radiosonde approach is typically restricted to about one thousand locations worldwide with 1 – 2 measurements per day. Water vapour radiometers are rather expensive instruments which require frequent calibration. GNSS receivers can measure the slant delay in the direction of a satellite, remove the contribution of the ionosphere and with some considerable post-processing estimate the system related errors (such as clock, orbit, phase centre) and obtain the total tropospheric delay. In order to arrive at vertical total delay (also called zenith total delay ZTD), the slant values are mapped to the vertical. To extract the water vapour content, the zenith hydrostatic delay (which can be obtained by measuring the pressure at the surface) is subtracted from the ZTD, giving the zenith wet delay (ZWD). The water vapour can be estimated from ZWD and the atmospheric temperature.
Credits
The information provided in this article has been compiled by GMV. In some cases, figures, tables and paragraphs have been extracted from the indicated references, in particular from the Galileo Science Opportunity Document.[26]
References
- ^ Springer, T., Gendt, G., Dow J.M., (Editors), “The International GNSS Services (IGS): Perspectives and Visions for 2010 and Beyond” Workshop 2006 Proceedings - Darmstadt, 2006
- ^ Calais, E., Minster, J. B., “GPS, Earthquakes, the Ionosphere, and the Space Shuttle, Physics of the Earth and Planetary Interiors”, Vol. 105, No. 3-4, 167-181, 1996.
- ^ Ducic, V., Artru, J. and Lognonne, P., “Ionospheric remote sensing of the Denali earthquake Rayleigh surface waves”, Geophysical Research Letters, Vol. 30, No. 18, pp. SDE 8-1, doi:10.1029/2003GL017812, 2003.
- ^ Jakowski, N., Wilken, V., Tsybulya K. and Heise, S., “Search of earthquake signatures by ground and space based GPS measurements”, in Flury, J., Rummel, R., Reigber, C., Rothacher, M., Boedecker, G., Schreiber, U. (Eds.) Observation of the Earth System from Space, Springer Berlin Heidelberg New York,ISBN: 3-540-29520-8, 2006.
- ^ Artru, J., Ducic, V., Kanamori, H., Lognonne, P., and Murakami, M., “Ionospheric detection of gravity waves induced by tsunamis”, Geophys. J. Int., Vol. 160, 840-848, 2005.
- ^ Hickey, M.P., Schubert, G. and Walterscheid R.L., “Propagation of tsunami-driven gravity waves into the thermosphere and ionosphere”, J. Geophys. Res., 114, A08304, doi:10.1029/2009JA014105.
- ^ Galvan, D.A., Komjathy, A., Mannucci, A., Hickey, M.P., Schubert, G., Walterscheid, R.L. and Occhipinti, G., “Towards Observing Tsunamis in the Ionosphere Using GPS TEC Measurements”, American Geophysical Union, Fall Meeting 2009, abstract #NH42A-03.
- ^ Martin-Neira, M., “A Passive Reflectometry and Interferometry System (PARIS): Application to Ocean Altimetry”, ESA Journal 1993, Vol. 17, pp. 331-355.
- ^ Garrison, J.L., Katzberg, S.J. and Hill, M.I., “Effect of sea roughness on bistatically scattered range coded signals from the Global Positioning System,” Geophys. Res. Lett., 25(13), pp. 2257–2260.
- ^ Zavorotny, V.U., Voronovich, A.G., “Scattering of GPS signals from the ocean with wind remote sensing application”, IEEE Transactions on Geoscience and Remote Sensing, 38(2), pp. 951-964, doi:10.1109/36.841977.
- ^ Beyerle, G. and K. Hocke, “Observation and simulation of direct and reflected GPS signals in Radio Occultation Experiments”, Geophys. Res. Lett., 28(9), pp. 1895–1898.
- ^ Cardellach, E., Ao, C.O., de la Torre Juárez, M., and Hajj, G.A., “Carrier phase delay altimetry with GPS-reflection/occultation interferometry from low Earth orbiters”, Geophys. Res. Lett., 31, L10402, doi:10.1029/2004GL019775.
- ^ Martin-Neira, M., Ruffini, G. and Buck, C., “Tsunami detection using the PARIS concept”, PIERS Online, 1(5), pp. 547-550, doi:10.2529/PIERS050217041500.
- ^ Buck, C and D’Addio, S: “Status and Perspectives of GNSS-R at ESA”, Proc IGARSS 2007, IEEE, ISBN: 978-1-4244-1211-2, pp. 5076-5079.
- ^ Ruffini, G and Soulat, F.: “On the GNSS-R Interferometric Complex Field Coherence Time”, Starlab Technical Brief TB 0005, 2008. http://arxiv.org/PS_cache/physics/pdf/0406/0406084v2.pdf
- ^ Masters, D., Zavorotny, V., Katzberg, S. and Emery, W., “GPS signal scattering from land for moisture content determination”, in: Proceeding of the IEEE International Geosciences and Remote Sensing Symposium, Honolulu, Hawaii, 24–28 July, pp. 3090–3092.
- ^ Wiehl, M., Legr'esy, B. and Dietrich, R. “Potential of reflected GNSS signals for ice sheet remote sensing”, J. Electromagnetic Waves and Applications, 17(7), pp. 1045-1047.
- ^ Komjathy, A., Maslanik, J.A., Zavorotny, V.U., Axelrad, P. and Katzberg, S.J., “Sea ice remote sensing using surface reflected GPS signals”, in: Proceedings of the IEEE International Geosciences and Remote Sensing Symposium (IGARSS 2000), Honolulu, Hawaii, 24–28 July, pp. 2855–2857.
- ^ Belmonte Rivas, M., Bi-Static Scattering of Global Positioning System Signals from Arctic Sea Ice, Ph.D. dissertation, University of Colorado, 2007.
- ^ Gleason, S.T., Hodgart, S., Yiping, S., Gommenginger, C., Mackin, S., Adjrad, M. and Unwin, M., “Detection and processing of bistatically reflected GPS signals from low-earth orbit, for the purpose of ocean remote sensing”, IEEE Trans. Geosci. Remote Sens., 43(6), pp. 1229-1241.
- ^ Pallarés J.M., Ruffini, G. and Ruffini, L., “Ionospheric tomography using GNSS reflections”, IEEE Trans. Geosc. Rem. Sens., 43(2), pp. 321-326.
- ^ Colombo O., Hernández-Pajares, M., Juan J.M., and Sanz, J. , “Wide-Area, Carrier-Phase ambiguity resolution using a tomography model of the ionosphere”, Journal of the Institute of Navigation, vol. 49, No.1, 2002.
- ^ Heise, S., Jakowski, N., Wehrenpfennig, A., Reigber Ch. and Lühr, H., “Sounding of the Topside Ionosphere/Plasmasphere Based on GPS Measurements from CHAMP: Initial Results”, Geophysical Research Letters, 29, No. 14, 10.1029/2002GL014738, 2002.
- ^ Jakowski, N., Wilken, V., Tsybulya K. and Heise, S., “Search of earthquake signatures by ground and space based GPS measurements”, in Flury, J., Rummel, R., Reigber, C., Rothacher, M., Boedecker, G., Schreiber, U. (Eds.) Observation of the Earth System from Space, Springer Berlin Heidelberg New York,ISBN: 3-540-29520-8, 2006.
- ^ Karabatic A., Weber R., Haiden, Th. and Leroch, S., “Near real-time estimation of tropospheric water vapour content from ground based GNSS data and its potential contribution to weather now-casting in Austria”, accepted for publication in Advances in Space Research.
- ^ Galileo Science Opportunity Document,